Hence the slope of the graph of the square function at the point (3, 9) is 6, and so its derivative at x = 3 is f ′ (3) = 6 More generally, a similar computation shows that the derivative of the square function at x = a is f ′ (a) = 2aF (x)=x^3 f (x)=\ln (x5) f (x)=\frac {1} {x^2} y=\frac {x} {x^26x8} f (x)=\sqrt {x3} f (x)=\cos (2x5) f (x)=\sin (3x) functionscalculator f\left (x\right)=x^3For example, if $f(x) = x^3 x 1$, $f^{1}(3) = 1$ because $f(1) = 3$ $\endgroup$ – Reese May 22 '18 at 1724 $\begingroup$ You would have to solve

Naszkicuj Wykres Funkcji H X 3 F X Funkcja F Na Zalaczonym Obrazku Prosze O Wytlumaczenie Jak Brainly Pl
F x x 3+e x/2
F x x 3+e x/2- Like the Function f (x)=x^3 Tom McCoy returns to bamboozle us And over what?Problem 324 Solution For x
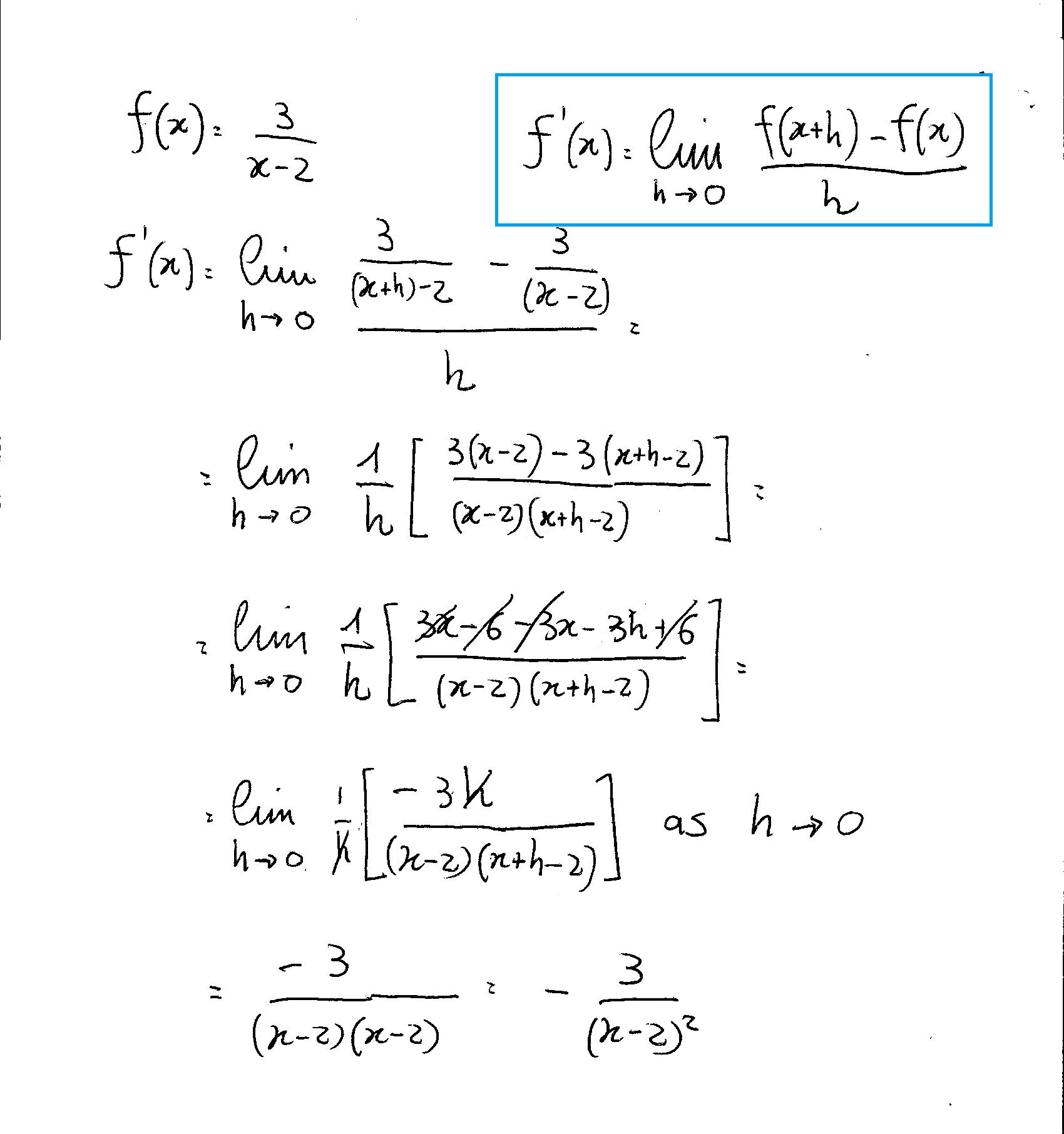



How Do You Find The Derivative Of F X 3 X 2 Using The Limit Definition Socratic
Misc 7 Find the intervals in which the function f given by f (x) = x3 1/𝑥^3 , 𝑥 ≠ 0 is (i) increasing (ii) decreasing f(𝑥) = 𝑥3 1/𝑥3 Finding f'(𝒙) f'(𝑥) = 𝑑/𝑑𝑥 (𝑥^3𝑥^(−3) )^ = 3𝑥2 (−3)^(−3 − 1) = 3𝑥2 – 3𝑥^(−4) = 3𝑥^2−3/𝑥^4 = 3(𝑥^2−1/𝑥^4 ) Putting f'(𝒙) = 0 3(𝑥^2− 1 Answer1 Active Oldest Votes 3 If your function is g ( x) = f ′ ( x 3), then it would be by the chain rule g ′ ( x) = f ″ ( x 3) 3 x 2 Otherwise, if you meant f ( x 3), it would be f ′ ( x) 3 xThe instructions are the same but the function is g (x) = 3x 5 x 3 The zeros are the points where 3x 5 x 3 = x 3 (3x 2 ) = 0 x = 0 is a zero three times and there are two others, x = ±√ (/3) For the remainder of the problem you need the derivative of g (x) The sign of the derivative tells us in the function is increasing
We set the denominator,which is x2, to 0 (x2=0, which is x=2) When we set the denominator of g (x) equal to 0, we get x=0 So x cannot be equal to 2 or 0 Please click on the image for a better understandingExtended Keyboard Examples Upload Random Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, history, geography, engineering, mathematics, linguistics, sports, finance, musicClick here👆to get an answer to your question ️ Domain and range of f(x) = x 3x 3 are respectively
Math131 Calculus I The Limit Laws Notes 23 I The Limit Laws Assumptions c is a constant and f x lim ( ) →x a and g x lim ( ) →x a exist Direct Substitution Property If f is a polynomial or rational function and a is in the domain of f, then = f x lim ( ) x aFind the Fourier series of f (x)= x^3 in x = Π to Π Watch later Share Copy link Info Shopping Tap to unmute If playback doesn't begin shortly, try restarting your device Up next3 The domain of the function f(x) = { ( x2 − 9) ( x − 3), if x ≠ 3 6 ifx = 3 is KEAM 16 4 Iff( x 1 2x − 1) = 2x, X ∈ N, then the value of is equal to f(2) is equal to KEAM 16 5 If n(A) = 5 and n(B) = 7, then the number of relations on A × B is



Dane Sa Funkcje F X 3 X Oraz G X F X Okreslone Dla Wszystkich Liczb Rzeczywistych X Zadania



Pochodna Funkcji F X X X 3
It is a different way of writing "y" in equations, but it's much more useful! In order to find what value (x) makes f (x) undefined, we must set the denominator equal to 0, and then solve for x f (x)=3/ (x2); Get an answer for 'f(x) = (x3)/(x1) find f'(0) f(x) = (x3)/(x1) find f'(0)' and find homework help for other Math questions at eNotes




Naszkicuj Wykres Funkcji F Podaj Wspolrzedne Zadanie Cwiczenie 3 Matematyka 1 Zakres Podstawowy Reforma 19 Strona 265



Cs Pwr Edu Pl Mydlarczyk R21z Rachprawd 21z Lz 03 Pdf
a) The function f(x) = 1/2x^4x^3x3 is continuous Now f(2) = 1 and f(25) = Since f(2) and f(25) have opposite signs, according to the intermediate value theorem, there is at least one root of f(x)=0 between 2 and 25 An interval like 2,25, where a continuous function takes different signs at the two endpoints, is called a bracketing interval In both theSo, the inverse of f(x) = 2x3 is written f1 (y) = (y3)/2 (I also used y instead of x to show that we are using a different value) Back to Where We Started The cool thing about the inverse is that it should give us back the original value When the function f turns the apple into a banana,Extended Keyboard Examples Upload Random Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, history, geography, engineering, mathematics, linguistics, sports, finance, music




S



Narysuj Wykres Funkcji F X X3 X2 2x
If f(x) = x3 \(\frac{1}{x^3}\)then show that f(x) f\((\frac{1}x)\) = 0 Welcome to Sarthaks eConnect A unique platform where students can interact with teachers/experts/students to get solutions to their queriesHence, the name "piecewise" function When I evaluate it at various x values, I have to be careful to plug theClick here to see ALL problems on Functions Question if f (x) = x^3, evaluate the difference quotient of (f (2 h) f (2)) / h and simplify Answer by solver () ( Show Source ) You can put this solution on YOUR website!



Powtorka Do Matury Matematyka Pracadomowa24 Pl




If F Mathbb R Setminus 0 1 To Mathbb R Satisfies F X 2f Left Frac 1 X Right 3f Left Frac X X 1 Right X Then 8f 4 Mathematics Stack Exchange
Summary "Function Composition" is applying one function to the results of another (g º f) (x) = g (f (x)), first apply f (), then apply g () We must also respect the domain of the first function Some functions can be decomposed into two (or more) simpler functionsA function is continuous if you can draw it without any stop ( without removing the pen) f(x) = {x^21,x=3 → 2ax at x=3 → 2a(3) = 6a if 8=6a then the function is continuous → if If I have a function f (x) = x^3 3x^2 3x 1 I would take the following steps Find the x intercepts for when y = 0 Find the y intercept when x = 0 Find the stationary points when dy\dx = 0 I would then have 2 x values from point 3 to plug into the original equation I would then draw a nature table do judge the flow of the graph or curve




Find The Average Rate Of Change Of F X X 3 3x From X1 2 To X2 0 Enotes Com



Wyznacz Dziedzine Funkcji F X X3 6x 9 Pod Pierwiastkiem Zaliczaj Pl
Given f(x) = 2x 3 and g(x) = –x 2 5, find (g o f)(1) When I work with function composition, I usually convert "(f o g)(x)" to the more intuitive " f (g(x))" form This is not required, but I certainly find it helpful In this case, I get (g o f)(1) = g(f(1))Use a comma to separate answers as needed Type each answer only once) The lesser zero of the function is of multiplicity so the graph off the xaxis atxThe greater zero of the function is of multiplicity so the graph of the xaxis at x = Analyze the polynomial function f(x) = x²(x 3)(x25) using parts (a) through (e) (Simplify your answerF(x) = x^3−2x^2−11x+12 Natural Language;



3 7 Graph Of Rational Functions Ppt Download



Wyznacz Pochodne Funkcji A F X 5x 2 7x 2 B F X 2x 3 X 1 Zaliczaj Pl
R > 0 then the function de–ned by f (x) = X1 n=0 c n (x a) n = c 0 c 1 (x a) c 2 (x a) 2 is di⁄erentiable (hence) continuous on (a R;aR) and 1 f0 (x) = c 1 2c 2 (x a)3c 3 (x a) 2 In other words, the series can be di⁄erentiated term by term 2 R f (x)dx = C c 0 (x a)c 1 (x a)2 2 c 2 (x a)3 3 In other words, theGraph {eq}f(x) = 3^x 1 {/eq} Graph The graph of an exponential function shows how rapidly the function increases as its input value increases Even for small changes in the input, the functionGiven the function f (x) as defined above, evaluate the function at the following values x = –1, x = 3, and x = 1 This function comes in pieces;



Let F R R Be A Function Such That F X X 3 X 2 F 1 Xf 2 F 3 X R Then F 2 Equals Sarthaks Econnect Largest Online Education Community



Prove That F X X 2 X 6 X 3 When X 3 5 When X 3 Is Continuous At X 3 Sarthaks Econnect Largest Online Education Community
Simple and best practice solution for f(x)=x^31 equation Check how easy it is, and learn it for the future Our solution is simple, and easy to understand, If f(x) = x3 \(\frac{1}{x^3}\), then show that f(x) f(\(\frac{1}{x}\)) = 0 Welcome to Sarthaks eConnect A unique platform where students can interact with teachers/experts/students to get solutions to their queries3 The function, f(x), passes through the point (10, 8) If f(x) is horizontally stretched by a scale factor of 5, what would be the new xcoordinate of the point?




Narysuj Wykres Funkcji F X 2 X 3 1 Brainly Pl
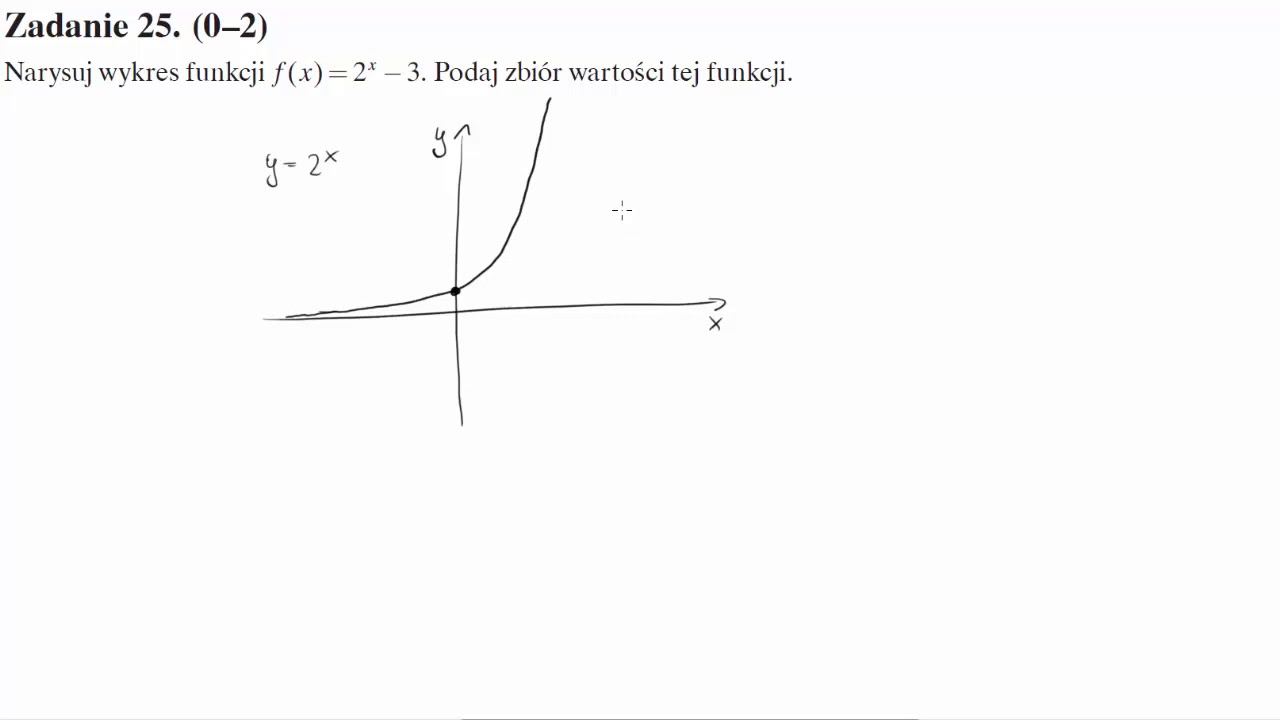



Matura Operon 17 Zadanie 25 Narysuj Wykres Funkcji F X 2 X 3 Podaj Zbior Wartosci Tej Funkcji Youtube
Ex 51, 7 Find all points of discontinuity of f, where f is defined by 𝑓(𝑥)={ (𝑥3, 𝑖𝑓 𝑥≤−3@ −2𝑥, 𝑖𝑓−3 This crossword clue Like the function f (x) = x^3 was discovered last seen in the at the New York Times Crossword The crossword clue possible answer is available in 5 letters This answers first letter of which starts with C and can be found at the end of C We think CUBIC is the possible answer on this clue21 Solve x 312 = 0 Add 12 to both sides of the equation x 3 = 12 When two things are equal, their cube roots are equal Taking the cube root of the two sides of the equation we get x = ∛ 12 The equation has one real solution This solution is x = ∛ 12 = 224 One solution was found x



F X X 4
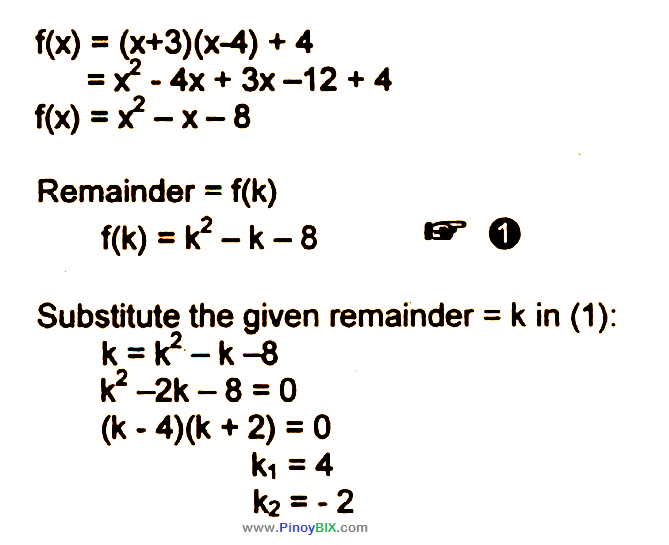



Solution Given F X X 3 X 4 4 When F X Is
To find the value of a function, f(x), simply put the value of x (this problem has x=3) into the expression For example, if f(x) = 2x 7 then f(3) = 2(3) 7Short Solution Steps f ( x ) = x ( 1 \frac { 4 } { x 3 } ) f ( x) = x ( 1 − x 3 4 ) To add or subtract expressions, expand them to make their denominators the same Multiply 1 times \frac {x3} {x3} To add or subtract expressions, expand them to make their denominators the sameF(x) = 13 4 x 3 2 x 2 has a xed point at x = 3=2, and f0(3=2) = 13 4 9 2 = 5=4, so it is repelling As we can see from the last two examples, changing a parameter (in this case a) can have the e ect of changing the situation from attracting to repelling Some



What Are The Domain And Range Of The Function F X 3 X 5 Solved
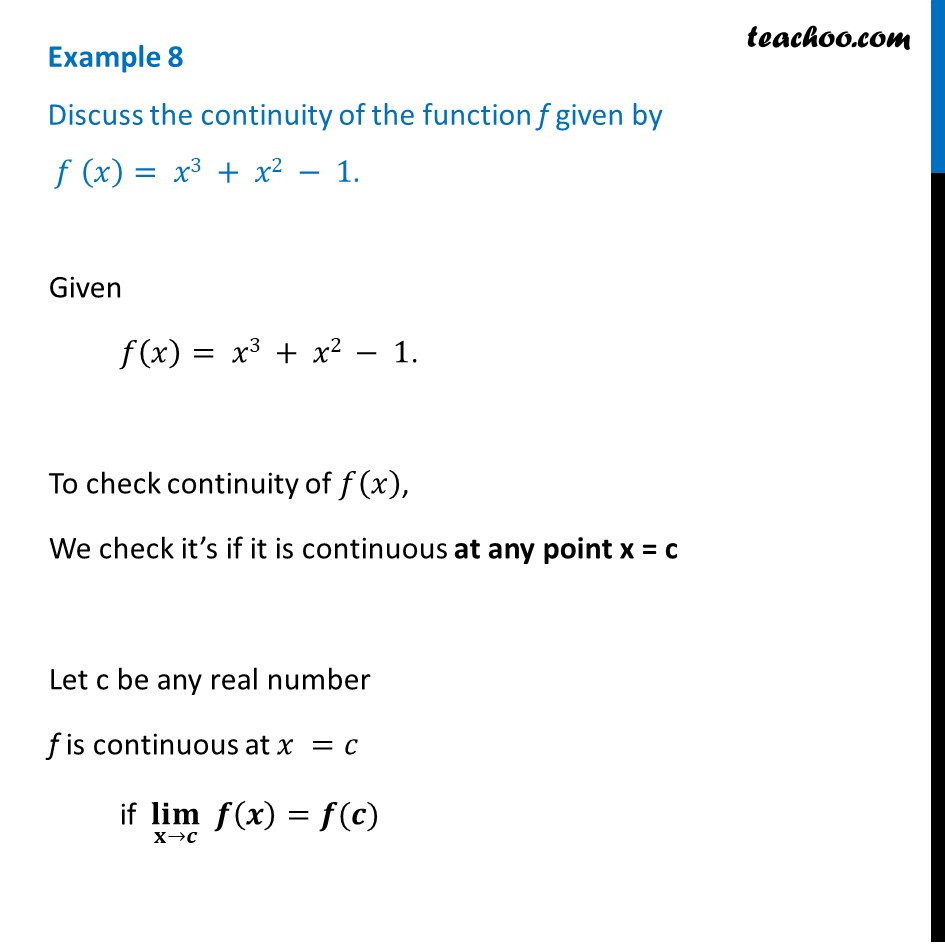



Example 8 Discuss Continuity Of F X X3 X2 1 Examples
Solution Steps f ( x ) = x ^ { 3 } 6 x 7 \text { at } x = 2 f ( x) = − x 3 6 x − 7 at x = 2 Consider the first equation Insert the known values of variables into the equation Consider the first equation Insert the known values of variables into the equation f\times 2=2^ {3}6\times 27 f × 2 = − 2 3Algebra Examples Popular Problems Algebra Find the Inverse Function f (x)=x^31Jose grade 11 student graph the exponential problem F(x)=3 x Hi Jose, Set up a table of values as you would for graphing other functions For example



Parent Functions




Liczba Miejsc Zerowych Funkcji F X X 1 X 3 Gdzie Xϵ Zadania Info Rozwiazanie Zadania Z Wartoscia Bezwzgledna
A teacher at a secondary school in London reviews equations on a whiteboard Credit Peter Macdiarmid, via GettySolution For If f ( x ) = \sqrt { x 3 } \sqrt { x 8 } then f ^ { \prime } ( x ) at x = 1 is Connecting you to a tutor in 60 seconds Get answers to your doubtsLet f (x) be a function defined in a domain D then the inverse of a function will exist if it is both oneone and onto The inverse of the function can find out by putting f (x)=y and then find



Funkcja Kwadratowa F Jest Okreslona Wzorem F X A X 1 X 3 Na Rysunku
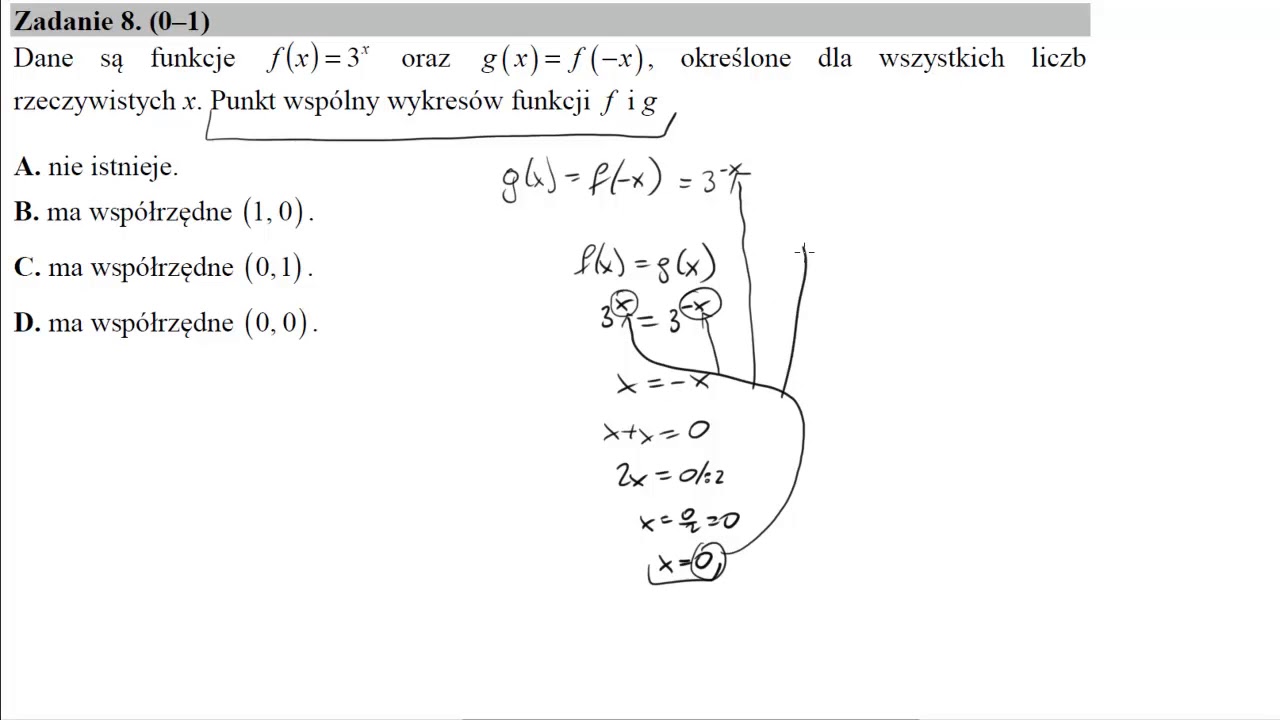



Dane Sa Funkcje F X 3x Oraz G X F X Okreslone Dla Wszystkich Liczb Rzeczywistych X Punkt Wspolny Wykresow Funkcji F I G
Solution for f(x)=x^39 equation Simplifying f(x) = x 3 9 Multiply f * x fx = x 3 9 Reorder the terms fx = 9 x 3 Solving fx = 9 x 3 Solving for variable 'f' Move all terms containing f to the left, all other terms to the right Divide each side by 'x' f = 9x1 x 2 Simplifying f = 9x1 x 24 The table of values for f(x) is shown below If g(x) is the result of f(x) being horizontally stretched by a scale factor of 3, construct its table of values and retain theFirst type the equation 2x3=15 Then type the @ symbol Then type x=6 Try it now 2x3=15 @ x=6 Clickable Demo Try entering 2x3=15 @ x=6 into the text box After you enter the expression, Algebra Calculator will plug x=6 in for the equation 2x3=15 2(6)3 = 15 The calculator prints "True" to let you know that the answer is right More Examples
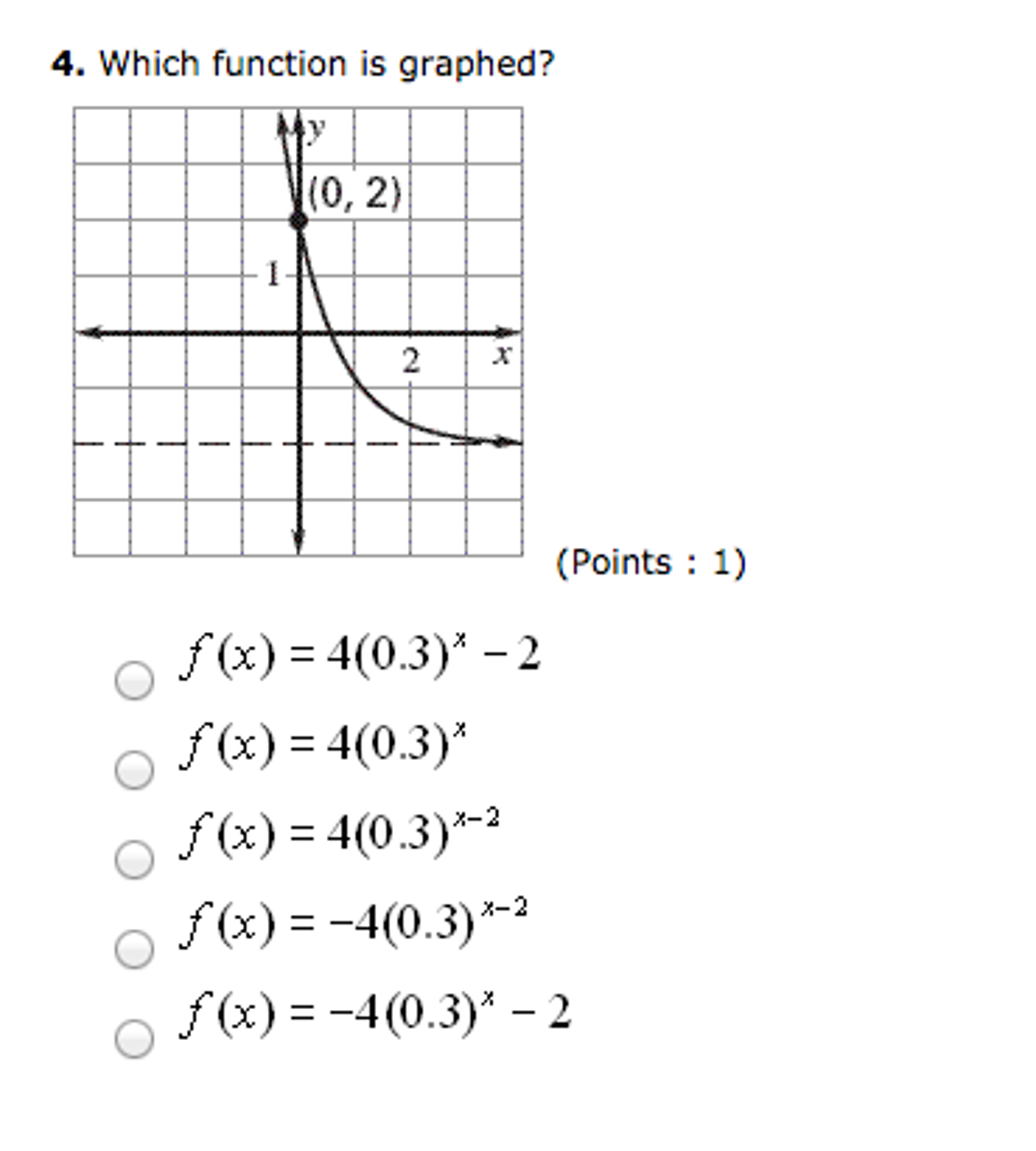



1 Find The Inverse Of The Function F X 3 5 Points Chegg Com




Prove That The Function Given By F X X3 3x2 3x 100 Is Increasing In R Mathematics Shaalaa Com
If x=3, f(3) = (1/2)^3 =1/8 If x=1, f(1) = (1/2)^1 =2 If x= 2, f(2) = (1/2)^2=4 If x=3, f(3) = (1/2)^3 =8 For the second equation, you meant to write this By the definition of logarithms, means So, make another table of values but in this case, start out with y=0, y=1, y=2, y=3, y=1, y=2, y=3 If y=0, then x = 2^0 =1 If y=1, thenThe function f(x) = x3 is increasing between 0 and 1 Therefore the supremum of the values on an interval (x i 1;x i) is f(x i) = x3i, and the in mum is f(x i 1) = x3i 1 Thus we can calculate the lower and upper sums of fwith respect to D n L(f;D n) = i=1 (i 1 n)3 1 n = 1 n4 i=1 (i 1)3 = 1 4n4 (n4 2n3 n2) n3 n4 = 1 4 1 2n 1 4n2 AJohn My calculator said it, I
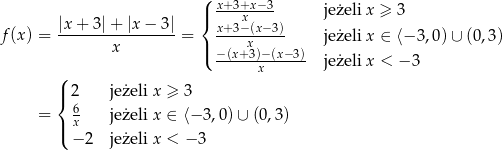



Dana Jest Funkcja F Okreslona Wzorem F X Frac X 3 X 3 X Zadania Info Rozwiazanie Zadania Wartosc Bezwgledna




Naszkicuj Wykres Funkcji F X 3 X X 1 Prosze O Dokladnie Rozwiazywanie Bez Omijania Nawet Brainly Pl
F (x)=x^3 prove\\tan^2 (x)\sin^2 (x)=\tan^2 (x)\sin^2 (x) \frac {d} {dx} (\frac {3x9} {2x}) (\sin^2 (\theta))' \sin (1) \lim _ {x\to 0} (x\ln (x)) \int e^x\cos (x)dx \int_ {0}^ {\pi}\sin (x)dx \sum_ {n=0}^ {\infty}\frac {3 Explanation First of all, let's compute the derivative of f (x), indicated as f '(x) f (x) = − x3 −3 ⇒ f '(x) = −3x2 In fact, to derive a sum you must derive each single term The first term is a power of x, and the derivative of xn is nxn−1 So, the derivative of x3 is 3x3−1 = 3x2, and since we had a minus sign in front of it, we will have to change signs the derivative of −x3 is −3x3−1 = −3x2Graph f (x)=x3 f (x) = x − 3 f ( x) = x 3 Rewrite the function as an equation y = x− 3 y = x 3 Use the slopeintercept form to find the slope and yintercept Tap for more steps The slopeintercept form is y = m x b y = m x b, where m m is the slope and b b is the yintercept y = m x b y = m x




Funkcja F Jest Okreslona Wzorem F X 2 X 2 1 X 3 2 Dla Kazdej Liczby Rzeczywistej X 2 Wartosc Funkcji F Dla Argumentu 2 Jest Rowna A 8 B 12 C 12 D 8



Openagh E Podreczniki Wypuklosc Funkcji Punkty Przegiecia




Naszkicuj Wykres Funkcji F X 1 X Zadanie 1 Matematyka 2 Zakres Podstawowy Po Gimnazjum Strona 46




Narysuj Wykres Funkcji F X 3x3 X Prosze O Wyjasnienie Brainly Pl
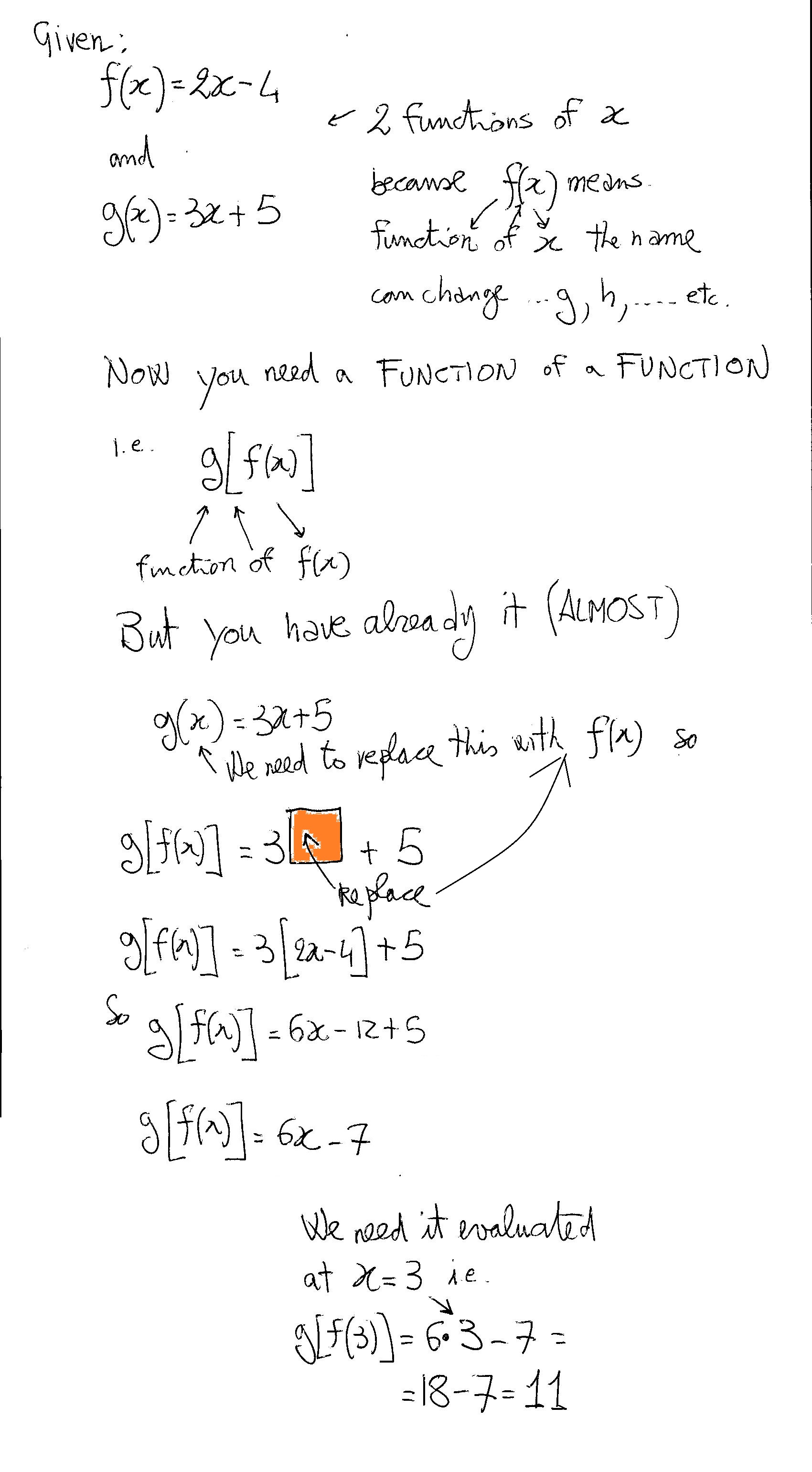



Given That F X 2 X 4 And G X 3 X 5 Find Gf 3 With Noob Like Steps Please I Need A Really Clear Working To Fully Understand Thanks 3 Socratic




Find The Domain And The Range Of The Real Function F X 1 X 3




Verifying Inverse Functions By Composition Not Inverse Video Khan Academy




If F X X 1 X 2 X 3 Then A Maximum Value Of F X Is 3 If X In 2 3 B Maximum Youtube




Interval Of F X Question Find The Intervals On Which F X 3x 4 4x 3 12x 2 2 Is My Working Out And Answer Correct Askmath



Jaka Jest Monotonicznosc Funkcji F X X 3 6x
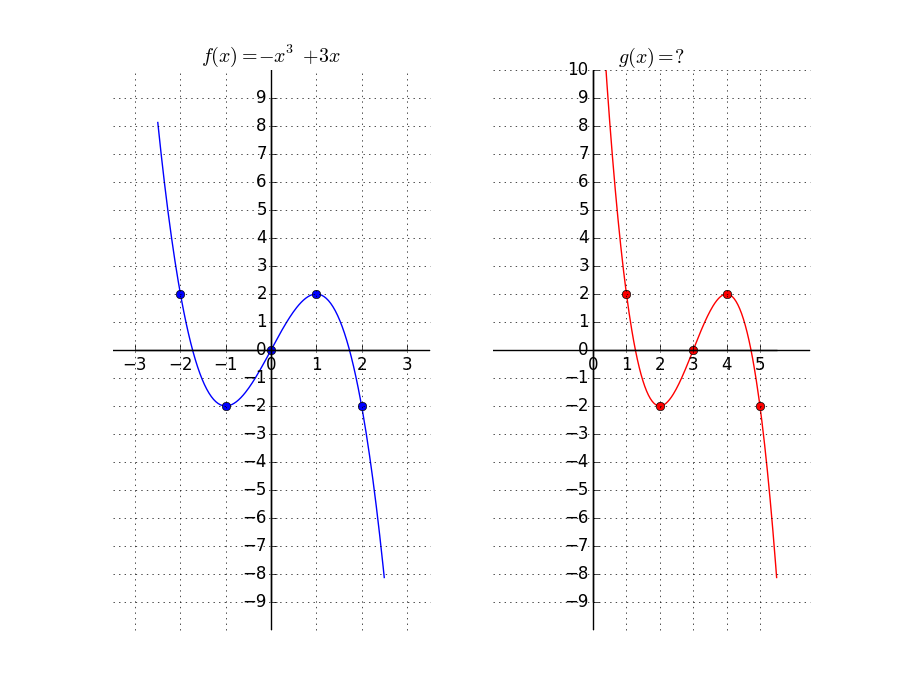



Given The Graph Of The Function F X X3 3x Find Chegg Com




If F X 3 4x 5 Then Find F 5 And F X Youtube
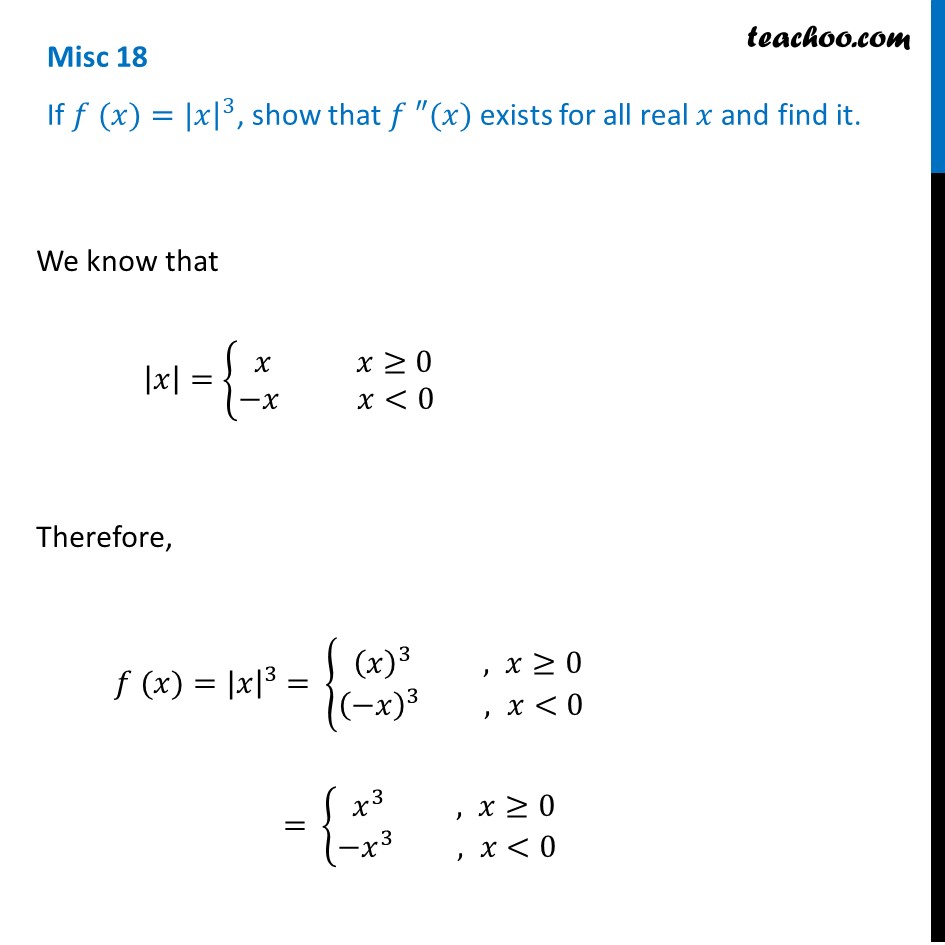



Misc 18 If F X X 3 Show That F X Exists And Find It
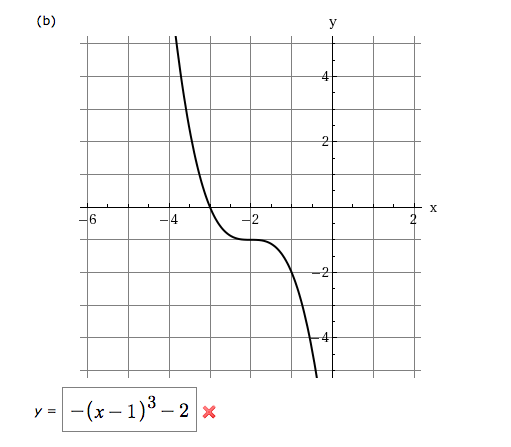



Use The Graph Of F X X3 To Write An Equation For Chegg Com



What Is The Solution Of Finding The Root Of The Equation F X X 3 2x 5 0 Initial Interval 2 3 Using The Newton Raphson Method Quora



Show That The Function F X X 3 X R Is Continuous But Not Differentiable At X 3 Sarthaks Econnect Largest Online Education Community
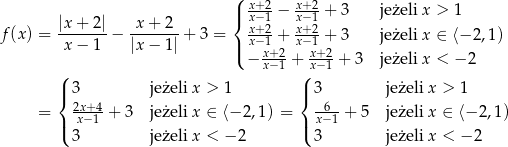



Funkcja F Jest Okreslona Wzorem F X Frac X 2 X 1 Frac X 2 X 1 3 Zadania Info Rozwiazanie Zadania Wartosc Bezwgledna



Zbiorem Wartosci Funkcji F X 2 X 3 Jest Przedzial A B Zadania Info Rozwiazanie Zadania Wykladnicza



Funkcja F Okreslona Jest Wzorem F X 3 X Funkcja G X F X 3 Z Prosta O Rownani



The Function F X X 3 X 1 F X X 2 4 3x 2 13 4 X 1 Is Sarthaks Econnect Largest Online Education Community



What Is The Point Of Inflection Of X 3 Quora
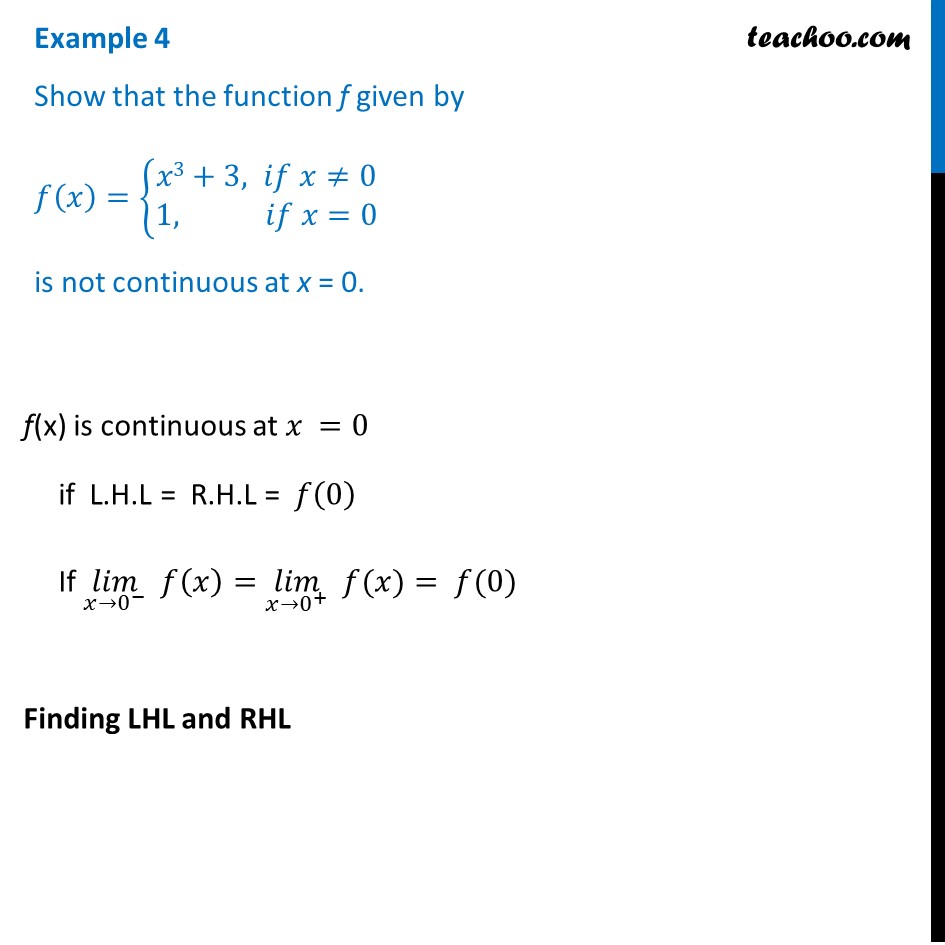



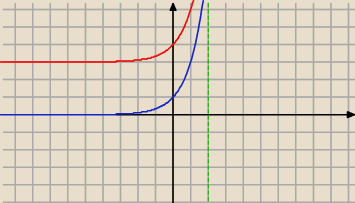
Example 4 Show F X X3 3 1 Is Not Continuous At X 0



Narysuj Wykres Funkcji F X 2 X 3 I Podaj Jej Zbior Wartosci Zadania Info Rozwiazanie Zadania Rysowanie Wykresu
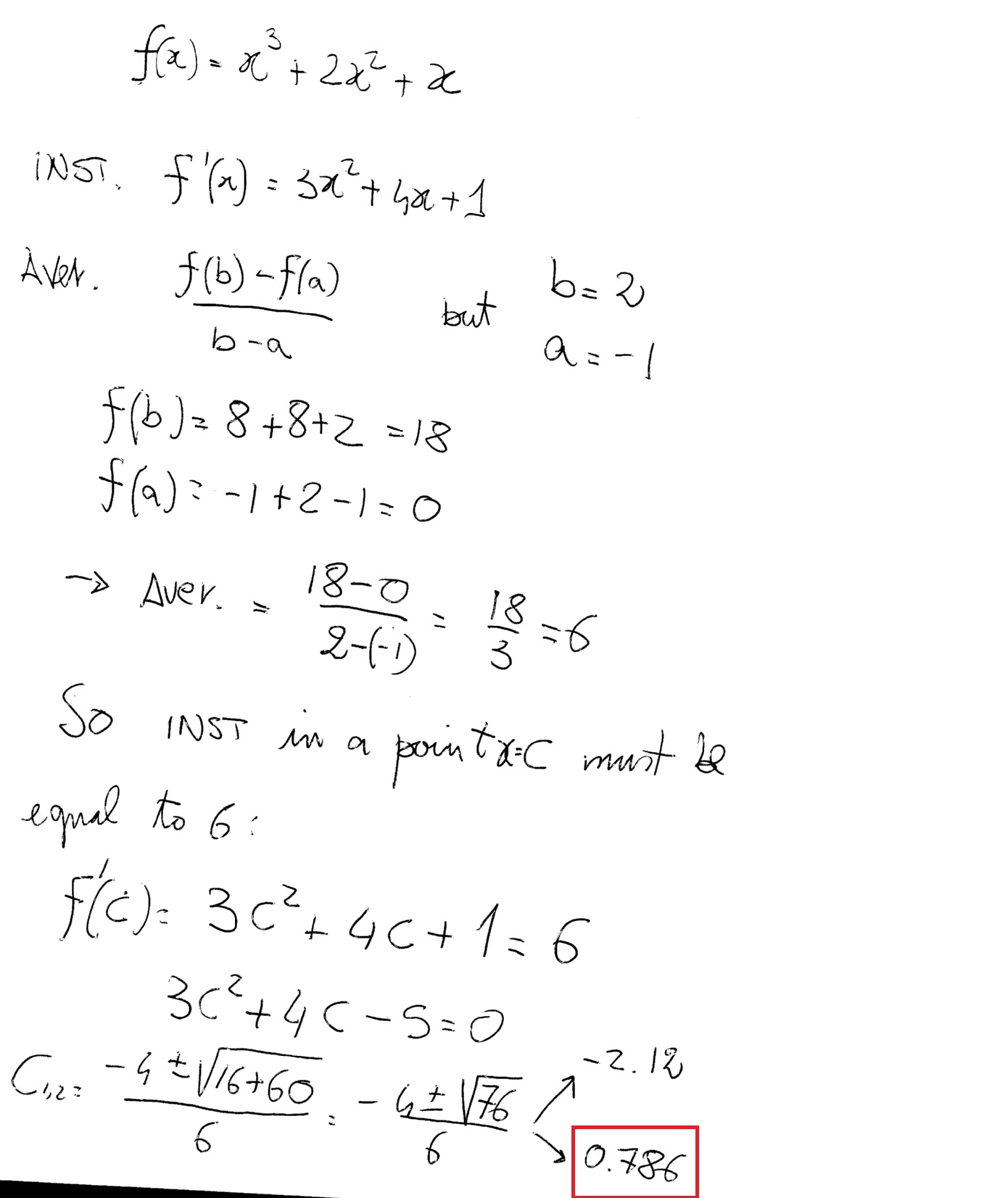



If F Is Defined By F X X 3 2x 2 X How Do You Find The Value Of X When The Average Rate Of Change Of F On The Interval X 1 To X
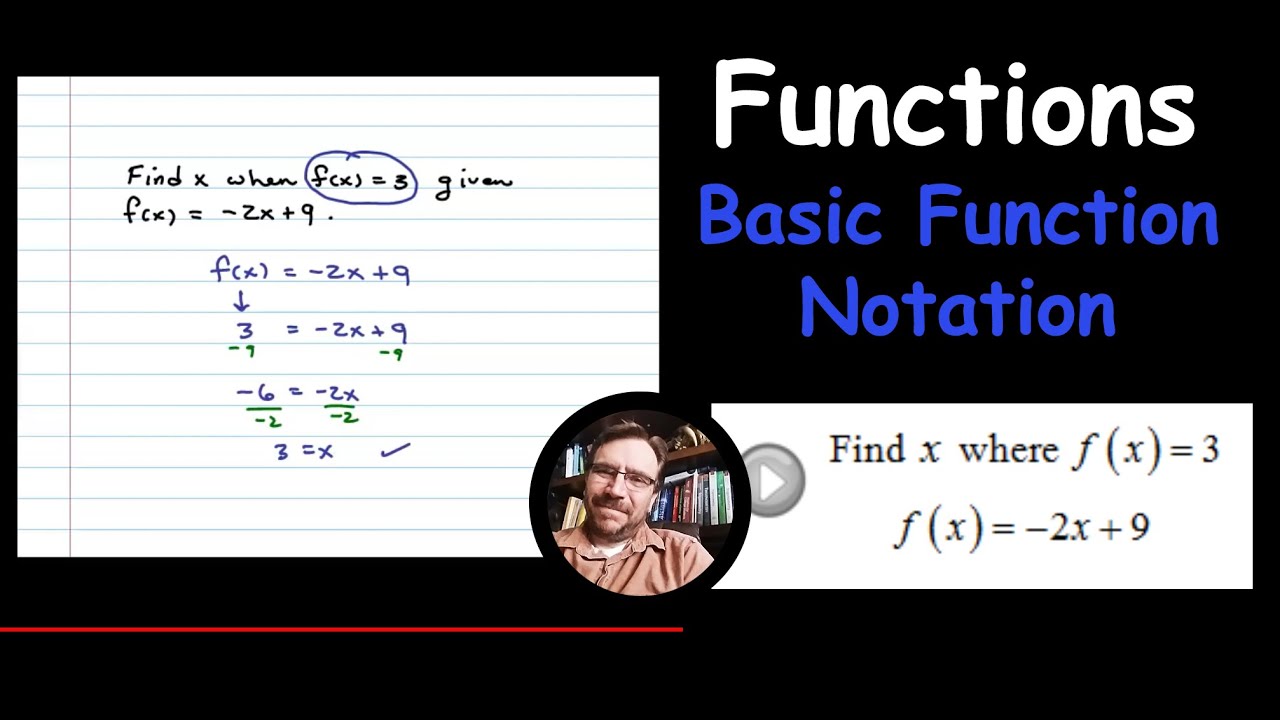



Evaluate Functions For X Given F X 3 Youtube
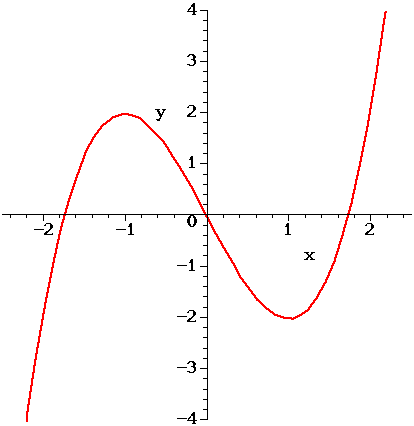



1 The Figure Above Shows The Graph Of F X X 3 Chegg Com
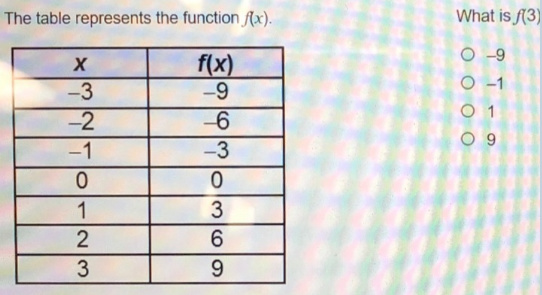



The Table Represents The Function F X What Is F 3 Home Work Help Learn Cbse Forum



Naszkicuj Wykres Funkcji F 3 X 2 Matematyka Pracadomowa24 Pl
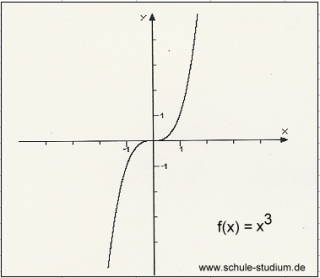



Funktionsgraphen Verschiedene Funktion X 3 X 4 Grafisch Dargestellt Schule Studium De Erklart Ausfuhrlich




Naszkicuj Wykres Funkcji H X 3 F X Funkcja F Na Zalaczonym Obrazku Prosze O Wytlumaczenie Jak Brainly Pl
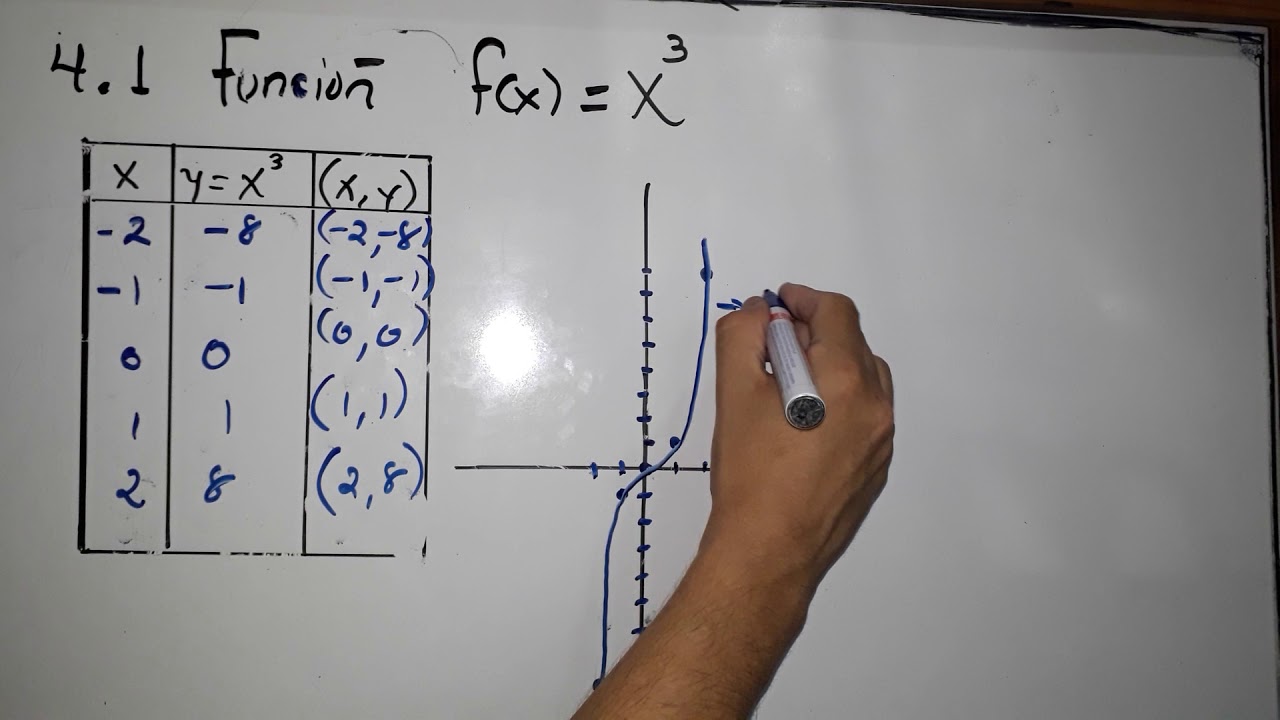



Funcion F X X 3 Youtube



It Is Given That For The Function F X X3 Bx2 Ax 5 On 1 3 Rolle S Theorem Holds With C 2 1 3 Find Values Of A And B Mathematics Topperlearning Com 3073



Function Definition Types Examples Facts Britannica




Ex 1 2 10 F X X 2 X 3 Is F One One Onto Class 12



Q Tbn And9gcrbi9blo Wofvbzwqyztuiacedgeo1l24bjehodcy52fa23rbjt Usqp Cau




If F X X 3 X 2 F 1 11 2 Then F X



Examine The Continuity Of The Function F X X3 2x2 1 At X 1 Studyrankersonline




Rozwiaz I Naszkicuj Wykres Funkcji F X X 3 X 1 Brainly Pl



Mat Ug Edu Pl Rlutowsk Pub Geog Zadania Pdf
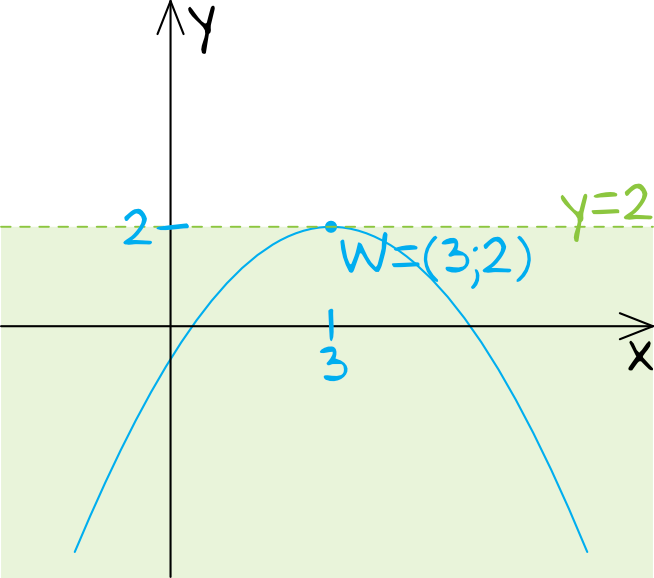



Wykres Funkcji F X 1 2 X 3 2 2 Ma Dwa Punkty Wspolne Z Prosta O Rownaniu Y M Jezeli Zadania
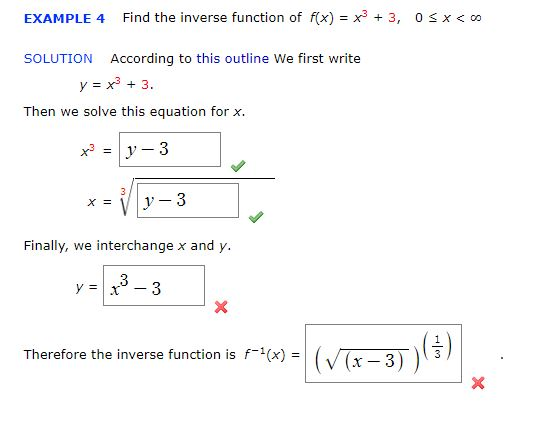



Find The Inverse Function Of F X X3 3 0 X Chegg Com




Show That The Function F X Modulus Of X 3 Is Continuous But Not Differentiable At X 3 Mathematics Topperlearning Com 8yq4x399




Narysuj Wykres Funkcji F X 3 X Brainly Pl



More Differentiation By First Principles



Q Tbn And9gcsmm4ktmxrxm10kmsg3wqo5rzire Ogwbm0v2tajixhbesgniod Usqp Cau
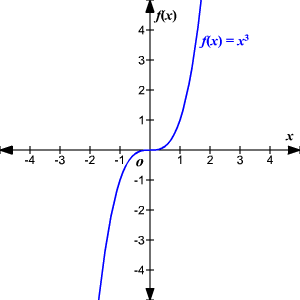



Even Odd Functions
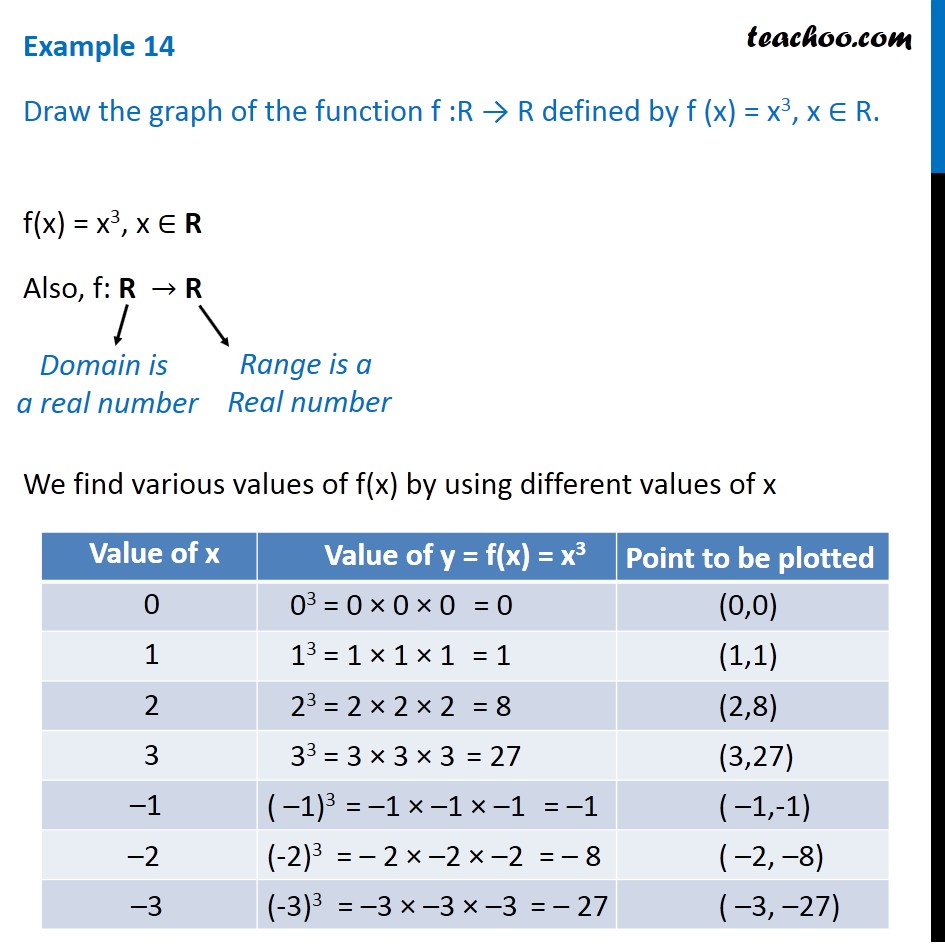



Example 14 Draw Graph Of F X X 3 Chapter 2 Class 11



Http Home Agh Edu Pl Konosek Wmn Ziip 1sem Zad Z02 Pdf



Www E Sgh Pl Niezbednik Plik Php Id Pid 2868
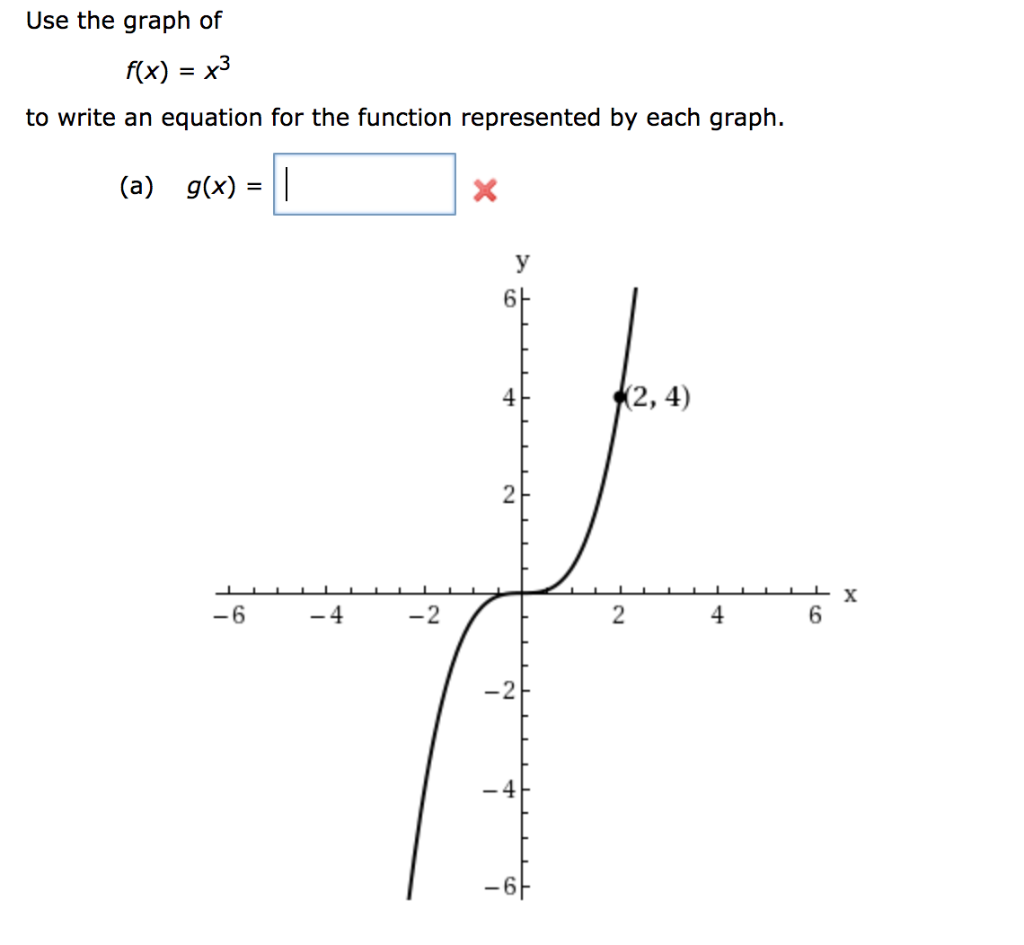



Use The Graph Of F X X3 Equation For The Chegg Com




Funkcja Kwadratowa F Jest Okreslona Wzorem F X A X 1 X 3 Wspolczynnik A We Wzorze Funkcji Zadania




Let F R R Be Given By F X X 2 3 Then F 1 Is Given By Sqrt X 3 B Sqrt X 3 C X Sqrt 3 D None Of These
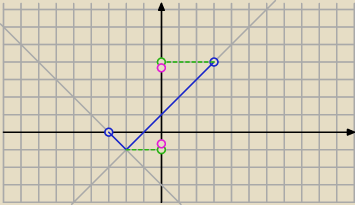



Zbiorem Wartosci Funkcji F X X 2 1 Dla 3 X 3 Jest



Biomath Functions




Naszkicuj Wykres Funkcji F X 2 X 3 2 Podaj 1 Dziedzine 2 Zbior Wartosci 3 Miejsce Zerowe 4 Brainly Pl
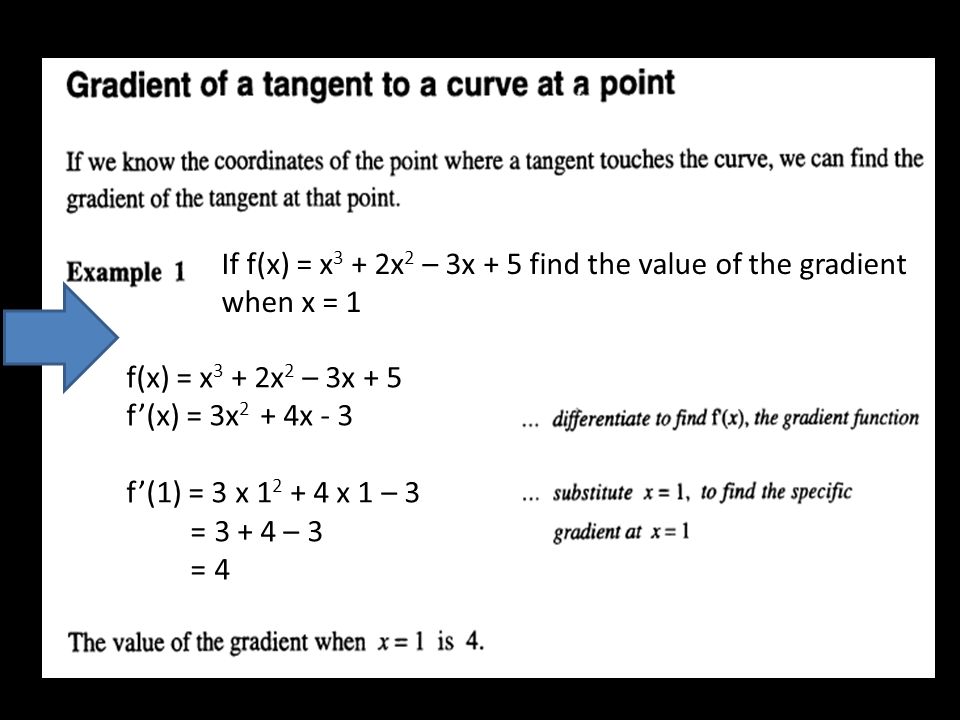



Differentiation F X X 3 2x 2 3x 5 F X 3x 2 4x 3 F 1 3 X X 1 3 3 4 If F X X 3 2x 2 Ppt Download




If F X X 3 Find The Value Of F 5 F 1 5 1



Narysuj Wykres Funkcji F X 2 X 3 Podaj Zbior Wartosci Tej Funkcji Zadania




Example 8 Find Derivative Of F X 3 At X 0 X 3 Examples



Funciones F X 3 X Geogebra




Let F X X 3 X 1 And Let G X Be Its Inverse Function Then Equation Of The Tangent To Y G X At X 3 Is



Http Www Uz Zgora Pl Esylwest Pliki An Mat Lista6 Ee Pdf



Graph Of F X X 3 X 0 X 10 Geogebra




The Relation F Is Defined By F X X 2 0 X 3 3x 3 X 10 The Relation G Is Defined By G X X 2 0 X 2 3x 2 X 10




How Do You Find The Derivative Of F X 3 X 2 Using The Limit Definition Socratic
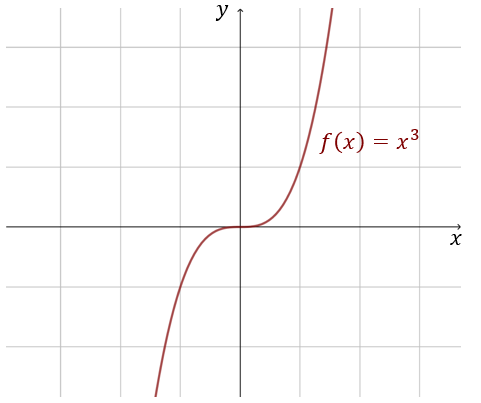



Funkcje Parzyste I Nieparzyste




Domain And Range Of F X X 3 X 3 Are Respectively




Zbiorem Wartosci Funkcji F X X 7 X 3 Jest Zadania




What Is The Difference Between F X X 2 1 And F X X 3 1 When Finding The Inverse Mathematics Stack Exchange




Consider The Function F X X 3 Draw The Graph Of F X Maths Relations And Functions Meritnation Com




Solution Given F X X 3 X 4 4 When F X Is Divided By X K The Remainder Is K Find K
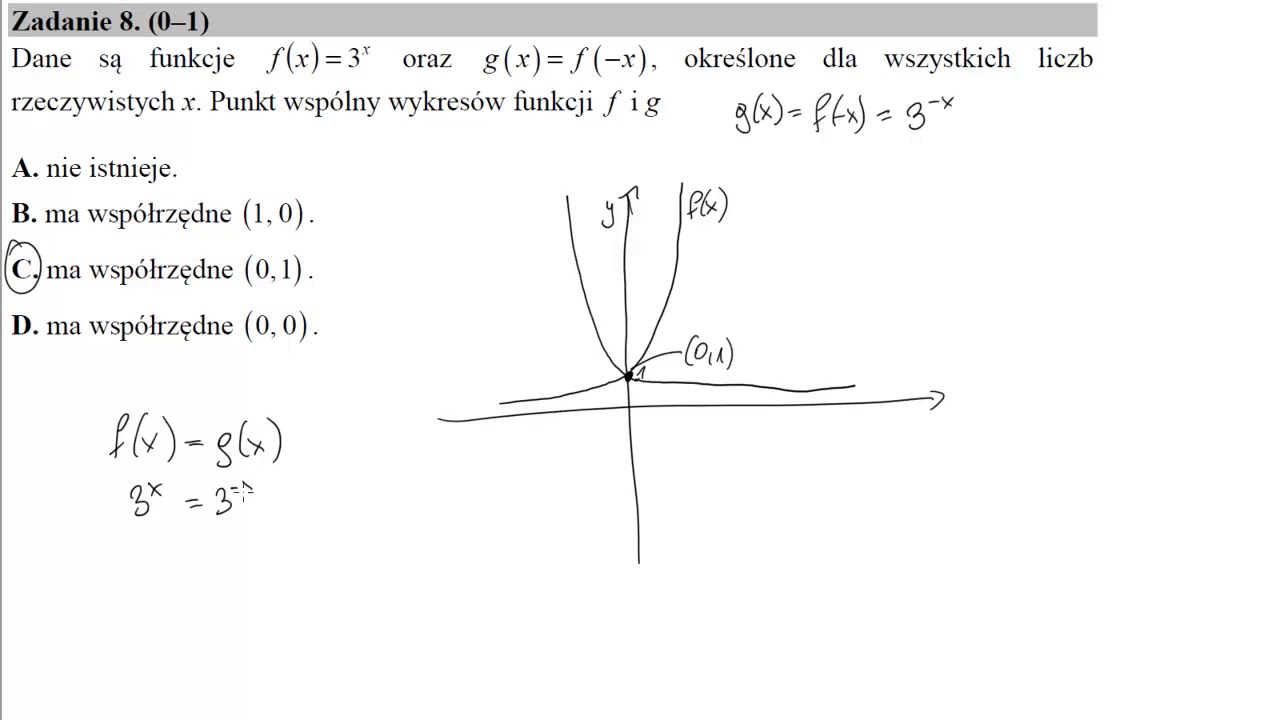



Dane Sa Funkcje F X 3x Oraz G X F X Okreslone Dla Wszystkich Liczb Rzeczywistych X Punkt Wspolny Wykresow Funkcji F I G A Nie Istnieje B Ma Wspolrzedne 1 0 C Ma Wspolrzedne 0 1 D Ma Wspolrzedne 0 0




Misc 7 Find Intervals F X X3 1 X3 X 0 Is Increasing




Consider F R 4 3 R 4 3 Given By F X 4x 3 3x 4 Show That F Is Bijective Find The Inverse Of F And Hence Find F 1 0



Funkcja F X 2x 5 X 3 Jest Malejaca



Funkcja F X X 3 12x Jest Przedzialami Monotoniczna



No comments:
Post a Comment